” Everything in our terrestrial world depends on the motions of electrons… “
Axel Becke
Quick introduction to ARPES
ARPES (Angle-Resolved Photoemission Spectroscopy) is an experimental method to study the motions of electrons. Light is used to eject electrons from the material and they are counted as a function of direction, photon- and kinetic energy.
Detected number of electrons can be plotted as a function of these four parameters in a number of ways:
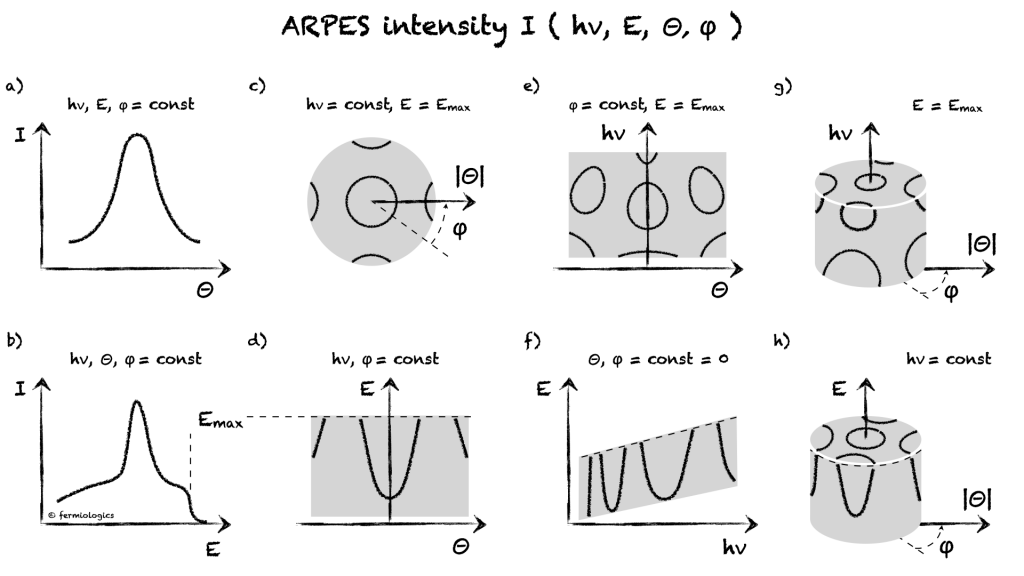
Such intensity distributions provide direct access to the Fermi surface and electronic structure of the studied material. They can be used to calculate physical properties, identify chemical composition or characterize the quality of the surface.
We offer a simple and direct way to detect electrons using our FeSuMa spectrometer.
From ARPES data to electronic structure of solids
The motions of electrons in the materials are best described by quantum mechanics, where they are treated like waves. Their crystal momentum is defined by the wave vector ( k ) whereas their energy ( EB ) is counted from the maximal possible energy of the electron in the crystal at zero temperature. Thus, electronic structure of the material can be represented by the probability to find electron in the state with a given (kx, ky, kz) and EB.
In order to relate ARPES intensity to this probability, one needs to find out how the crystal momentum and energy of the electron in the solid change after absorption of photon and transmission through the surface. Energy EB is typically determined by subtracting experimentally determined cutoff energy Emax from kinetic energy.
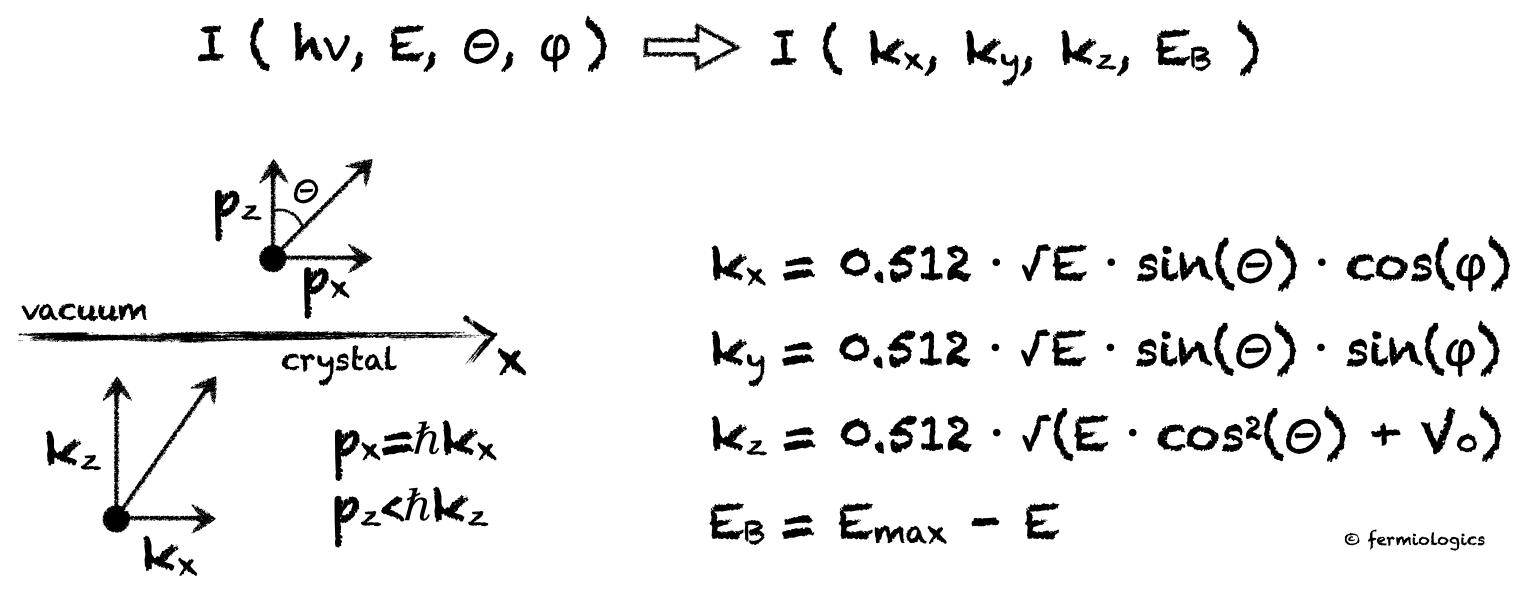
Momentum behaves similar to the optical refraction: parallel to the surface component of the wave vector is conserved, and perpendicular component can be calculated assuming a free-electron-like state of electron in the crystal after absorbing the photon.
Figure below shows which portions of momentum-energy space are probed by ARPES datasets.
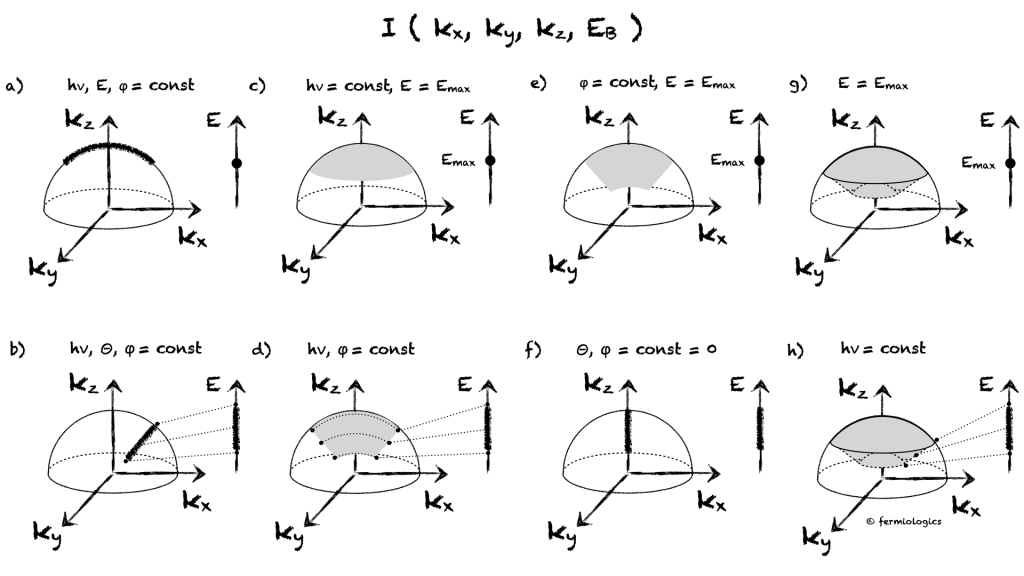
If the energy is kept constant, the interpretation of the ARPES intensity is relatively simple. The top row of panels shows that the angular distributions only have to be recalculated in the momentum coordinates and, for example, the distorted contours of the Fermi surface become symmetrical in momentum space. On the contrary, the lower row of panels shows that the interpretation of the energy distributions is not trivial. For example, the widely used energy distribution curve (panel b) also corresponds to the range of momenta from the radius of the momentum sphere and is therefore not as intuitive as the angular distribution from panel a).
If only Fermi-electrons are of interest, the usual nowadays energy-momentum cuts (panel d) probe only an arc on the IkI=const sphere, whereas 2D angular detection, as in FeSuMa, probes the whole spherical dome (panel c).
